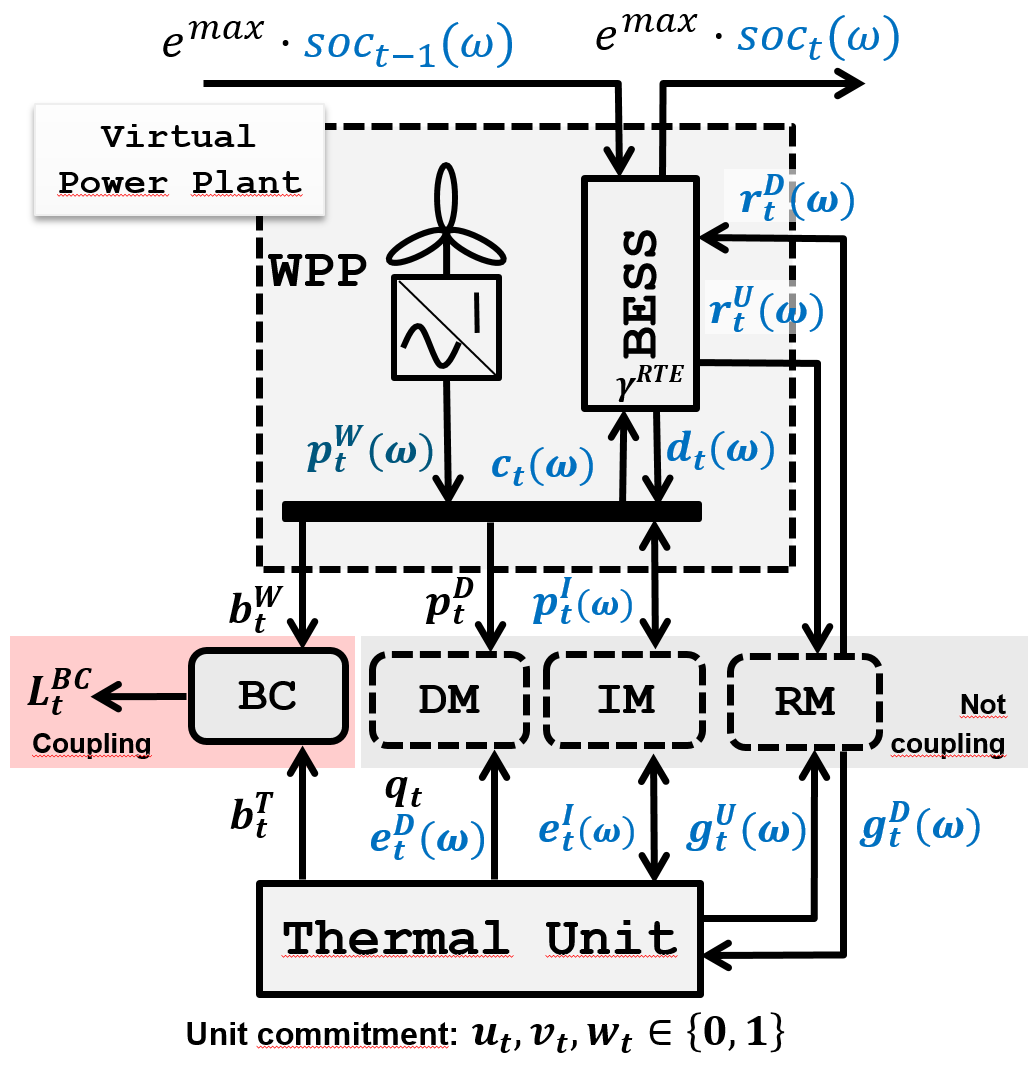
The research project Modelling and Optimization of StruCtured problems and Applications (MOSCA) of the GNOM research group of the UPC has been granted by the Agencia Estatal de Investigación (AEI , Government of Spain) in the call PROYECTOS DE GENERACIÓN DE CONOCIMIENTO 2022. The goal of this project is to develop new optimization methods for structured problems, and its application to real cases. I participate as IP, together with professor Jordi Castro, and my specific interest in the project is to work on the formulation and efficient optimization of a multistage stochastic programming model for the optimal participation of energy communities in electricity multi-markets, continuing the research of the ongoing project OptiREC.
